
Charlie and Liz have each started nibbling at their chocolate bars.
They bump into each other in the park and this is what they have:

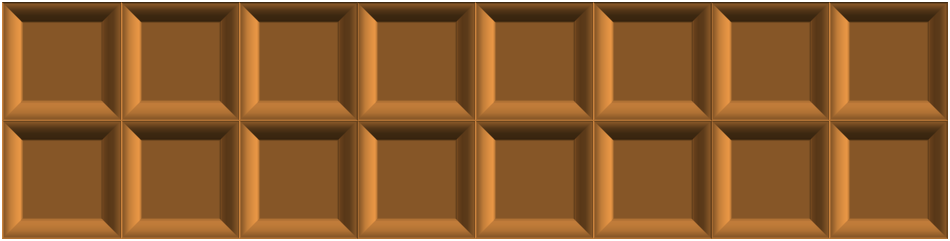
Charlie has 16 squares. Liz has 10 squares. Would they be able to share them equally?
The following week, Charlie and Liz meet again. Here is the chocolate they plan to share this time:
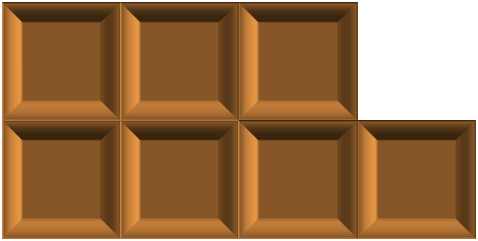
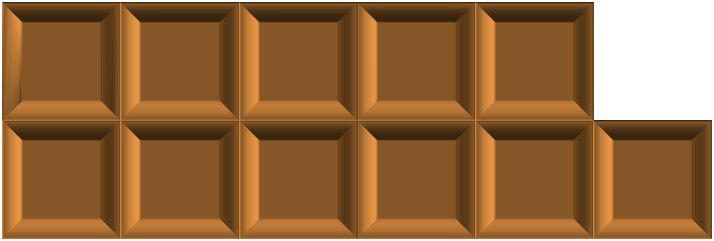
Would they be able to share their chocolate equally this time?
In the weeks that follow, Charlie and Liz meet up regularly at the park. Here are the numbers of squares of chocolate they have each time they meet:
9 and 5
4 and 7
12 and 10
5 and 6
When will they be able to share their chocolate equally?
Can you create an image (diagram) that helps you decide?
If Liz and Charlie are sharing a large number of Lego bricks, creating an image to represent all the bricks will take too long...
Can you create simple diagrams to help you decide if they will be able to share their bricks equally, if
- Liz had 41 and Charlie had 72 bricks?
- Charlie had 4 621 457 and Liz had 659 201 bricks?!
Can you explain how you decide if any two large numbers of bricks can be shared equally?
You might now like to try Chocolate for Three
