Folding Fractions

Can you fold a piece of paper in half? Of course you can, it’s easy, you just match the two corners along one side. But can you fold it in thirds? You might be able to fold it into thirds with a bit of fiddling and guessing, but what about into fifths? Or sevenths? Or thirteenths? Here is a simple way you can fold a piece of paper into any fraction you would like – exactly – no guessing or fiddling needed!

Folding a third

To start, take a square piece of paper and mark half way along top side with a small crease.

Now fold the bottom left corner to meet this halfway mark and crease the paper.

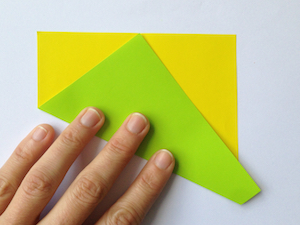
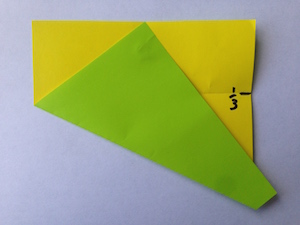
You should now see two triangles (yellow in our picture) that have been created with the folded paper. Mark half way along the right-hand side of the triangle on the right with a small crease (by matching the top right corner to where the top layer of paper crosses the bottom). This mark is 1/3 of the length of the side of your square piece of paper. Try it out and check for your yourself!

Folding any fraction

Kazuo Haga, a retired biology professor from Japan, came up with this ingenious method. Although a biologist, he was very interested in using origami to explore mathematics (you can find our more in his fascinating book). In fact, Haga realised that this method was even more useful.

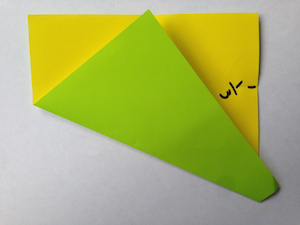
We've already seen that starting with the half-way mark at the top of the square paper allowed us to fold a third. What if we rotate the paper and fold the bottom left corner up to a point 1/3 along the top edge?

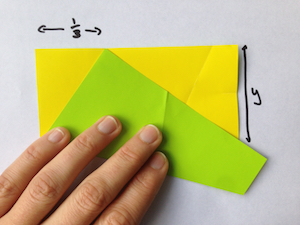
This time, the half-way mark along $y$, the right side of the right triangle, is 1/4 of the length of the side of your square paper.

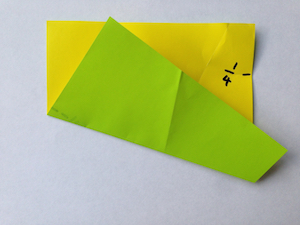
So far, starting from 1/2, we've folded 1/3, and starting from 1/3 we've folded a quarter. And if we carry on repeating Haga's method we can fold every unit fraction (one with 1 in the numerator)!

Folding 1/5....

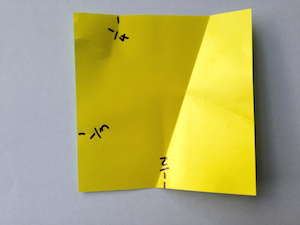
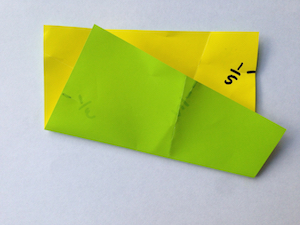
... folding 1/6....

...folding 1/7...

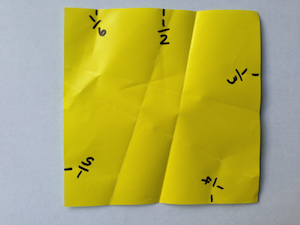
...and so on. And then we can use these to create any multiple of these unit fractions, and so fold any fraction. Hooray for Haga!

(You can find out why this works in Folding fractions – explained.)