
Here is a picture of a chess board:
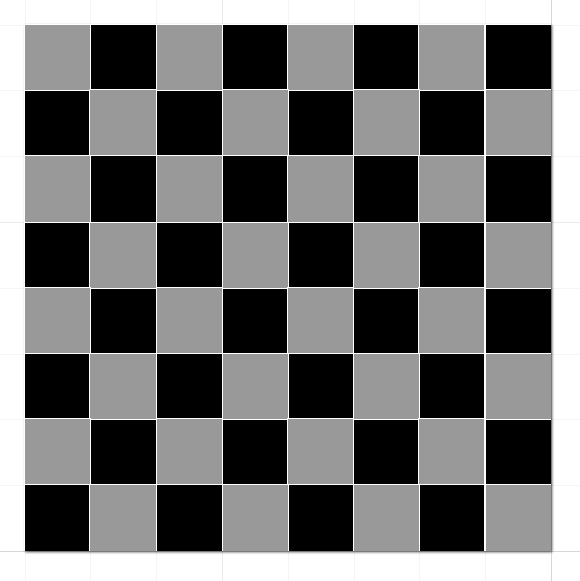
How many squares can you see altogether?
Once you've had a go, here are some questions you might like to consider:
- Are all your squares the same size? (I can see some that are bigger than others...)
- How many different sizes of square are there?
- How many squares are there of each size?
- Would it help to start by counting the squares on a smaller board first?
- Is there a quick way to work out how many squares there would be on a 10x10 board? Or 100x100? Or...?
- What about a rectangular chessboard?
Comments
Answer
There are 64 blocks which are all the same size. All you had to do was 8 times 8 which equals 64 because it is aboard that is 8 by 8.
- Log in to post comments
Maths / Chessboard squares
I also see there are 64 squares ('cause 8 x 8 is 64). However, all the squares have the same size. Why? Well, I measured it with a ruler and they all have the same size. Sometimes, our eyes see illusions instead of the reality. Check it.
- Log in to post comments
Mathematics / Chessboard
Luisa saw that there were bigger squares because the question is "How many squares are there?" But it doesn't clarify what type of squares, so there are bigger and smaller squares, meaning, there are more than 64 squares. The bigger squares are composed by smaller squares. So a big square would have 4 mini small squares. (Bigger ones could have more :) )
PS: If a question is posted by Cambridge, well we can guess it won't be some very easy questions. :)
- Log in to post comments
Chessboard Challenge
The answer is 204 squares, because you have to add all the square numbers from 64 down.
- Log in to post comments
represent each type of square
represent each type of square as a letter or symbol ,and use that as a quick way to work out how many of each type of square.
- Log in to post comments
answer
you can work this out by drawing 8 separate squares, and on each find how many squares of a certain size are there. For 1 by 1 squares there are 8 horizontally and 8 vertically so 64.For 2 by 2 there are 7 horizontally and 7 vertically so 49 . For 3 by 3 there are 6 and 6, and so on and you find that after you have done that for 8 by 8 you can go no more so add them up and find there are 204.
- Log in to post comments
Interesting...
There are actually 64 small squares, but you can make bigger squares, such as 2 times 2 squares
- Log in to post comments
chessboard challenge
we have predicted that there are 101 squares on the chessboard. There are 64 1 by 1 squares,28 2 by 2 squares,4 4 by 4 squares,4 6 by 6 squares,1 8 by 8 square ( the chessboard)
- Log in to post comments
answer strategy
The answer is 204.
My method: If you take a 1 by 1 square you have one square in it. If you take a 2 by 2 square you have 4 small squares and 1
2 by 2 square. In a 1 by 1 square the answer is 1 squared, in a 2 by 2 square the answer is 1 squared + 2 squared in a 3 by 3 square the answer is 1 squared + 2 squared + 3 squared, etc. So in an 8 by 8 square the answer is 1 squared + 2 squared
+ 3 squared + 4 squared + 5 squared + 6 squared + 7 squared + 8 squared which is equalled to 204.
- Log in to post comments
Chess board challenge
There are 165 squares because there are 64 of the tiniest squares and 101 squares of a different bigger size, combining the tiniest squares into the bigger ones.
- Log in to post comments
Total 204 squares
Total 204 squares
8×8=1
7×7=4
6×6=9
.
.
.
.
.
.1×1=64
Total204
- Log in to post comments
My solution
I came to the conclusion that the answer is 204.
Firstly, I worked out that there were 64 'small squares' on the chess board.
The next size up from the 1x1 would be 2x2 squares.Since there are 8 rows and columns, and there is an 'overlap' of one square for each of these, there are 7 2x2 squares on each row and each column, so there are 49. What I mean by overlap is how many squares longer by length each square is than 1.
For 3x3 squares, there is an overlap of 2, and so there are 8 - 2 squares per row and column, and therefore 6x6 of these, which is 36.
For 4x4 squares, the overlap is 3, so there are 5 per row and column, leaving 25 squares.
This is repeated for all other possible sizes of square up to 8x8 (the whole board)
5x5: 16
6x6: 9
7x7: 4
8x8: 1
64+ 49 + 36 + 25 + 16 + 9 + 4 + 1 = 204.
Interestingly, the quantities of the squares are square numbers which decrease as the size of the square increases - this makes sense as the larger the square, the less likely there is going to be sufficient space in a given area for it to fit. It also makes sense that the quantities are square numbers as the shapes we are finding are squares - therefore, it is logical that their quantities vary in squares.
Thanks! Kevin Gu
- Log in to post comments
answer
it is 204 because you add all the square numbers from 64 down to 1 and end up with the answer of 204
- Log in to post comments
Chessbord Answer
The answer is 204 squares. This is because you have to calculate how many 1 x 1 squares, 2 x 2 square, 3 x 3 squares and so on that are on the chessboard. These numbers end up being the square numbers: 64, 49, 36, 25, 16, 9, 4, 1. These added together equals 204.
For a larger chessboard such as a 10 x 10 board your answer would be 385 because you add all the square numbers from 1 - 10.
- Log in to post comments
I get 204 squares because you
I get 204 squares because you know where the top corners of each square are to fit the size eg. 2x2 squares must have a top corner at least one row above and to the left of the bottom left, 3x3 must be be two above. So using this you can work out that there is 64 1x1s, 49 2x2s, 36 3x3s ect. You can see the pattern of square numbers appearing and then you can add them all up to get a final total of 204
- Log in to post comments
Chessboard Challenge, Most Systematic Approach.
We found that there were 204 squares altogether on the chessboard. This is how we did it.
First of all we counted how many 8x8 squares there were (which wasn't very hard as there was one).
Next, we conted how many 7x7 squares there were; which there were 4, one in every corner. We did this until we finished counting the 1x1 squares. We also found out that the nxn was a square number as how many squares of that size fitted into the chessboard there were. (These were all the results for how many squares there were starting at 8x8:
8x8=1 square
7x7=4 squares
6x6=9 squares
5x5=16 squares
4x4=25 squares
3x3=36 squares
2x2=49 squares
1x1=64 squares)
We hope you'll respond posotivly and we hope we are correct.
Thank you for reading this comment.
- Log in to post comments
Perhaps better to start with 2x2 and then 3x3 chessboard
I started with the 10x10 chessboard as suggested but I think it'd be easier to start with 2x2 and then 3x3, getting bigger. This will help find the general rule.
So, for 2x2 chessboard:
1st question to ask: How many 1x1 squares?
2nd question to ask: How many 2x2 squares?
Now go on to a 3x3 chessboard.
Express each answer in relation to the size of the original chessboard.
- Log in to post comments
Let's start by using this
Let's start by using this strategy and listing out how many squares there are in 1x1, 2x2 etc:
1x1: 1
2x2: 5
3x3: 14
From this, we can see that we are adding on square numbers, 4 and then 9. But how to explain this?
Well, imagine a 4x4 square. The quantity of a type of square, for example 2x2, in this larger square can be expressed in the form (n-x)^2 where n is the length of the large square. The n-x part explains how many you can fit onto one row/column. Of course, due to the squares taking up more than a single square, their quantity is less than n, and this explains the x part: a 2x2 square will have an x value of 1 because it takes up less space than a 3x3, which will have an x value of 2 (the larger the x value, the fewer you can fit onto one row/column)
Since it is a chessboard, the measure of how many you can fit onto each row and column needs to be squared to see exactly how many you can fit in total. Since we are squaring this, the result will always be square, hence the reason why square numbers are being added on.
But why do the square numbers increase?
Well, it can be explained by the increasing size of the square itself. You see, the expressions (n-x)^2 remain the same for a type of square you are trying to find, e.g. the number of 2x2s can be found by (n-1)^2, or the number of 4x4s by (n-3)^2. As n increases, a new type of square can be found, and therefore a new (n-x)^2 expression is added on. For example, take a look at a 3x3 vs a 4x4:
3x3 = n^2 + (n-1)^2 + 1
4x4 = n^2 + (n-1)^2 + (n-2)^2 + 1
There is a new (n-2)^2 part because there is now more than one 3x3, so there needs to be a way to find it out.
What you will notice, however, is that despite the (n-1)^2 parts both looking for 2x2s, they have different values when n increases - this makes sense as if the size of the square increases, you'd expect to find more of a certain type of square. The (n-2)^2 part of the 4x4 instead has the same value as the (n-1)^2 part in the 3x3. From this, we can see that the square numbers have 'shifted over' - that each time, a larger square number is added on because all of the other square numbers are now represented by the other (n-x)^2 parts.
I therefore have proved that as the size of the square increases, the square numbers added on increase.
The sequence (of number of squares) therefore follows the pattern:
1,5,14,30,55,91,140,204,285
We can see that the 8th number in the sequence is 204, so 204 is how many squares an 8x8 will have.
But a general rule? Well, if we allow n to be the length of the side of the big square, the number of squares is equal to:
n^3/3 + n^2 /2 + n/6.
This is the nth term for this particular sequence, and so serves as a way of quickly working out the number of squares in any chessboard, such as 338350 squares in a 100x100
By Kevin Gu, Wilson's School
- Log in to post comments
Answer
the correct answer is 96 cause u get:
- 64 1x1 squares
- 15 2x2 squares
- 7 3x3 squares
- 4 4x4 squares
- 2 5x5 squares
- 1 6x6 square
- 1 7x7 square
- 1 8x8 square
if you sum all of those totals the answer is 96.
- Log in to post comments
How to email
Hi there, I have a solution on a word document, so is there an email address I can send it to? I don't want to use the link above as I don't have outlook configured on my PC.
Thanks
- Log in to post comments
Mathematics (chessboard)
The numbers on the side and along the top of a chessboard go down from 8 all the way down to 1, to find the total number you square the numbers e.g 8 squared , 7 squared, etc. until you get to 1,then add all of your answers together also the main square surrounding the chessboard and you will find that the answer equals 204. The works because if you were to find out how many 2x2 squares there were you will find how many squares in a row you can fit and you will find as you go up in squares the number you can fit in each row goes down for example 8 squared 64, 7 squared is 49. Also if you had 10x10 you would get 100 extra squares, it doesn't matter how big the chess board is if you square the number going across and continue going down squaring the numbers then add them you will get the amount of squares.
- Log in to post comments
chessboard
we have found that the squares overlap. if you use 8 square numbers then you find the total you find the amount of squares
1. 64
2.49
3.36
4.25
5.16
6.9
7.4
8.1
add them all together and the total number of squares are 204 !!!
- Log in to post comments
Answer and Solution Chessboard challenge
My answer is 204, and here is the solution (easy way):
Add all square numbers up till 8x8
8x8 = 64
7x7 = 49
6x6 = 36
5x5 = 25
4x4 = 16
3x3 = 9
2x2 = 4
1x1 = 1
Now add them all together which gives 204.
- Log in to post comments

